![]() Even though options trading may seem complicated to new traders, Joseph Burgoyne, Director, Institutional and Retail Marketing, for The Options Industry Council, provides helpful guidance to understanding options pricing.
Even though options trading may seem complicated to new traders, Joseph Burgoyne, Director, Institutional and Retail Marketing, for The Options Industry Council, provides helpful guidance to understanding options pricing.
To be a successful options investor, it's important to understand how options prices move and what causes those moves. All too often, new options traders are left baffled as to why an options contract languishes while its underlying stock takes off. Thankfully, guidance can be found in pricing models and the Greeks. Simply stated, the Greeks are a group of mathematical models that each help to calculate the theoretical value of an option. Here we will introduce two of the Greeks, delta and theta.
D (Delta)
There is not necessarily a direct linear relationship between an option's value and the price of the underlying equity. As such, each option has a delta that describes the theoretical relationship between the two. Delta expresses how far the value of an option is likely to move based on a $1 move in the price of the underlying stock, and is expressed as a range between zero and 1.00. The higher the delta, the closer the price moves of the option will mimic those of the underlying. For example, if an option has a delta of .90 and the underlying moves $1, then the option contract would move $0.90, barring no other factors.
A helpful illustration for the concept of delta is an adult walking with a child. If an adult is walking with a three-year old, for every step the adult takes, the child can only take one-third of the gait. The child is an out-of-the-money option and only has a delta of .33. If the child instead is 10 years old, he's now an at-the-money option with a delta of .50 since he now can cover half the gait of the adult. Finally, as he or she grows up and becomes stock, or adult-like, for every adult step, he or she moves at the same rate and is now a deep-in-the-money option.
? (Theta)
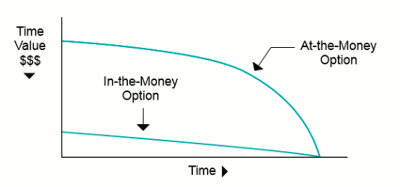
Options have two stores of value - intrinsic and time. Only in-the-money options have intrinsic value and its calculation can be consistently defined as the difference between the stock price and strike price, while time value has several variables and decreases as the option approaches expiration. This decrease is known as time decay, and its rate is measured by theta.
Theta is not a constant, rather the decay of an option's value accelerates as the option approaches expiration. An at-the-money option's value will accelerate to zero as it approaches expiration.
Essentially, theta tries to account for the unknowns of the future. Although anything can happen during the life of an option, the chance of a dramatic move happening drops significantly as its expiration date draws close.
Theta can be particularly important for options on a stock, index or ETF that is not moving very much as it expresses how much value that option will lose every day or week by owning that option if the underlying price is unchanged. Conversely, if you are short the option, then theta tells you how much you would make if it remains unchanged. So, generally, Theta hurts buyers and helps sellers of options.
Although other factors can affect an option's price, understanding delta and theta can remove a lot of the mystery for retail traders. Come back next month when we discuss income generation strategies using options.
DISCLAIMER: OPTIONS INVOLVE RISK AND ARE NOT SUITABLE FOR ALL INVESTORS. INDIVIDUALS SHOULD NOT ENTER INTO OPTIONS TRANSACTIONS UNTIL THEY HAVE READ AND UNDERSTOOD THE RISK DISCLOSURE DOCUMENT CHARACTERISTICS AND RISKS OF STANDARDIZED OPTIONS AVAILABLE BY VISITING WWW.OPTIONSEDUCATION.ORG.
ANY STRATEGIES DISCUSSED, INCLUDING EXAMPLES USING ACTUAL SECURITIES AND PRICE DATA, ARE STRICTLY FOR ILLUSTRATIVE AND EDUCATIONAL PURPOSES ONLY AND ARE NOT TO BE CONSTRUED AS AN ENDORSEMENT, RECOMMENDATION OR SOLICITATION TO BUY OR SELL SECURITIES. PAST PERFORMANCE IS NOT A GUARANTEE OF FUTURE RESULTS. COPYRIGHT C 2016 THE OPTIONS INDUSTRY COUNCIL. ALL RIGHTS RESERVED.
By Joseph Burgoyne, Director, Institutional and Retail Marketing, for The Options Industry Council