Demystifying Delta in Option Trading

In this article, I will provide an example of using Delta as an approximate measure of probability.

Online Trading Academy offers a two-day option course in which the five Greek option components are discussed in depth. One of the slides in the introduction option curriculum is called “Three Views of Delta.” Each of the three points is defined in the following way. The first: As a measure of the change in an option's value with respect to a change in the price of the underlying; the percentage of any stock price change which is directly related in the option price. The second: As a hedge ratio; Delta tells how many underlying shares of the options are required for a neutral hedge. The third and last: As an approximate measure of probability; how much of a chance does the option have of expiring ITM (in the money).

At first glance, reading the content of this slide leaves a lot of novice options students completely puzzled. Many times they even comment that the verbiage used to define the Delta sounds foreign (or Greek) to them. In the class, I do have the luxury of time to elaborate on each of those three points until the point is clear to all present. Our classes are usually not greater than 24 students.

The goal of these articles on the topic of Delta is to elaborate on each of these three "views of Delta." In my previous article, “Delta as a Hedge,” I gave the intricate explanation of the Delta as a hedge ratio (the second view of Delta). I have no intention of repeating myself; hence, the link is provided above for the readers who have not had the chance to read that newsletter. Moreover, the Delta as a measure of the change in an option's value with respect to a change in the price of the underlying (the first view of delta) has also been explained in detail in an article I wrote last year. In this article, I have in fact also pointed out how closely the Delta and Gamma are related.

Now, let me demystify the third view of Delta, an approximate measure of probability. The previously quoted definition states, "Delta as an approximate measure of probability spells out how much of a chance does the option have of expiring ITM (in the money).

To make this point clearer, let me break it into pieces. Every market transaction is composed of two parties: A buyer and a seller. If an option has a delta of 0.70, and the buyer purchases that option, then the buyer has a 70% chance of seeing that option expire ITM (in the money). At the same time, the other side, the seller who has sold that option with a delta of 0.70, has only a 30% chance of seeing this option expiring out of the money, and therefore, worthless, allowing him to keep the premium he received for selling the option. This point is visually presented in Figure 1 below.

Click to Enlarge

Whether the option is bought or sold does not change the delta percentage. What really matters is at what side of the table are we on as the option traders. Once again, in our classes, we emphasize the fact that when selling options, the implied volatility (IV) should be high and the strike price selection should be done on the delta value. If, for instance, we have sold the delta of 0.30, it would mean that there is only a 30% chance of probability of that option ever being in the money. The very reason why we would even consider selling the option is because we would like to see the option decay in premium and expire worthless. The very last thing that we would like to see as the option seller is the increase in the value of the premium that we have sold, receiving payment up front.

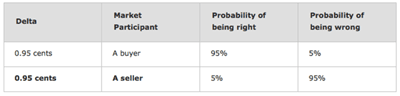
Now, let us presume that we accidentally have ignored the rule of selling OTM (out-of-the-money) options with low delta values and have proceeded with the sale of a 0.95 delta. In that case, the situation would look like this:

Click to Enlarge

Lastly, let me emphasize the importance of language precision. Delta, as an approximate measure of probability, spells out how much of a chance the option has of expiring ITM (in the money); yet, it does not specify by how much it will be ITM. If it will be ITM at expiry, the exact amount depends on the price of the underlying.

In conclusion, in this article, the final one on the topic of Delta, I have provided the classroom definition of three views of Delta. For the first two definitions, the links to my previous articles were provided. However, for the third view, the specific explanation is laid out. Delta is not the only Greek component of options, so I might revisit in the near future other components such as Vega and Theta. As always, I suggest constant learning and the expanding of option knowledge. Trade options wisely based on your intricate knowledge of options.